How Approximate Anchored Value Iteration Handles Errors in Decision-Making Models
Approximate Anchored Value Iteration (Apx-Anc-VI) is shown to be robust against Bellman operator evaluation errors, offering performance comparable to standard Approximate VI.
:::info Authors:
(1) Jongmin Lee, Department of Mathematical Science, Seoul National University;
(2) Ernest K. Ryu, Department of Mathematical Science, Seoul National University and Interdisciplinary Program in Artificial Intelligence, Seoul National University.
:::
1.1 Notations and preliminaries
2.1 Accelerated rate for Bellman consistency operator
2.2 Accelerated rate for Bellman optimality opera
5 Approximate Anchored Value Iteration
6 Gauss–Seidel Anchored Value Iteration
7 Conclusion, Acknowledgments and Disclosure of Funding and References
5 Approximate Anchored Value Iteration
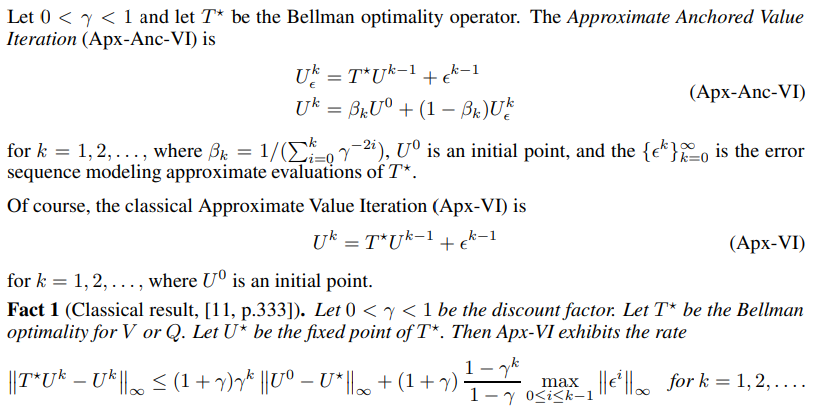
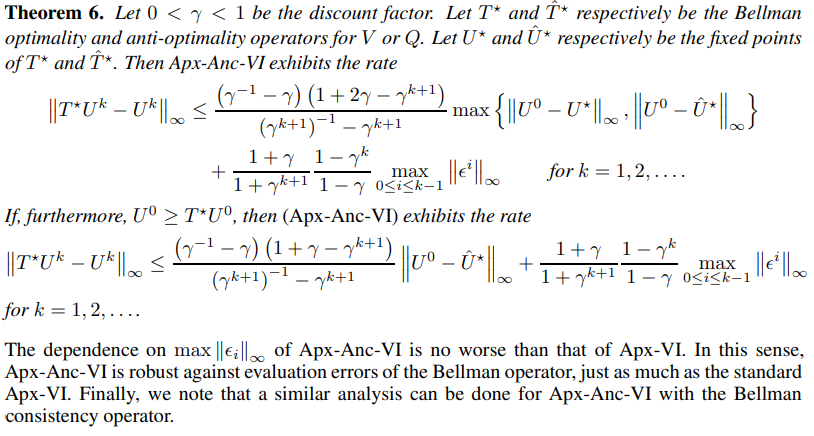
In this section, we show that the anchoring mechanism is robust against evaluation errors of the Bellman operator, just as much as the standard approximate VI.
\
\
\
:::info This paper is available on arxiv under CC BY 4.0 DEED license.
:::
\
What's Your Reaction?









































































































